Prove Continuity of Reciprocal Function by Composites F1f2 F1f2
Reciprocal Function
A reciprocal function is obtained by finding the inverse of a given function. For a function f(x) = x, the reciprocal function is f(x) = 1/x. The reciprocal function is also the multiplicative inverse of the given function. The reciprocal function can be found in trigonometric functions, logarithmic functions, and polynomial functions.
Let us learn more about reciprocal functions, properties of reciprocal functions, the graph of reciprocal functions, and how to solve reciprocal functions, with the help of examples, FAQs.
| 1. | What Are Reciprocal Functions? |
| 2. | Properties of Reciprocal Functions |
| 3. | How To Graph Reciprocal Functions? |
| 4. | Domain and Range Of Reciprocal Functions |
| 5. | How To Solve Reciprocal Functions? |
| 6. | FAQs on Reciprocal Functions |
What Are Reciprocal Functions?
For a given function f(x), the reciprocal is defined as \( \dfrac{a}{x-h} + k \), where the vertical asymptote is x=h and horizontal asymptote is y = k . The reciprocal function is also called the "Multiplicative inverse of the function".
The common form of a reciprocal function is y = k/x, where k is any real number and x can be a variable, number or a polynomial. The reciprocal of a number is a number which when multiplied with the actual number produces a result of 1 For example, let us take the number 2. The reciprocal is 1/2. Also, when we multiply the reciprocal with the original number we get 1
\(\begin{align} \dfrac{1}{2} \times 2 = 1\end{align}\)
Some examples of reciprocal functions are, f(x) = 1/5, f(x) = 2/x2, f(x) = 3/(x - 5). In the exponent form, the reciprocal function is written as, f(x) = a(x - h)-1 + k.
Properties Of Reciprocal Functions
The reciprocal functions can be easily identified with the following properties.
- Reciprocal functions are in the form of a fraction. A numerator is a real number and the denominator is either a number or a variable or a polynomial.
- The reciprocal of x is 1/x.
- The denominator of a reciprocal function cannot be 0. For example, f(x) = 3/(x - 5) cannot be 0, which means 'x' cannot take the value 5.
- The domain and range of the reciprocal function f(x) = 1/x is the set of all real numbers except 0.
- The graph of the equation f(x) = 1/x is symmetric with the equation y = x.
How To Graph Reciprocal Functions?
There are many forms of reciprocal functions. One of them is of the form k/x. Here 'k' is real number and the value of 'x' cannot be 0. Now let us draw the graph for the function f(x) = 1/x by taking different values of x and y.
| x | -3 | -2 | -1 | -1/2 | -1/3 | 1/3 | 1/2 | 1 | 2 | 3 |
| y | -1/3 | -1/2 | -1 | -2 | -3 | 3 | 2 | 1 | 1/2 | 1/3 |
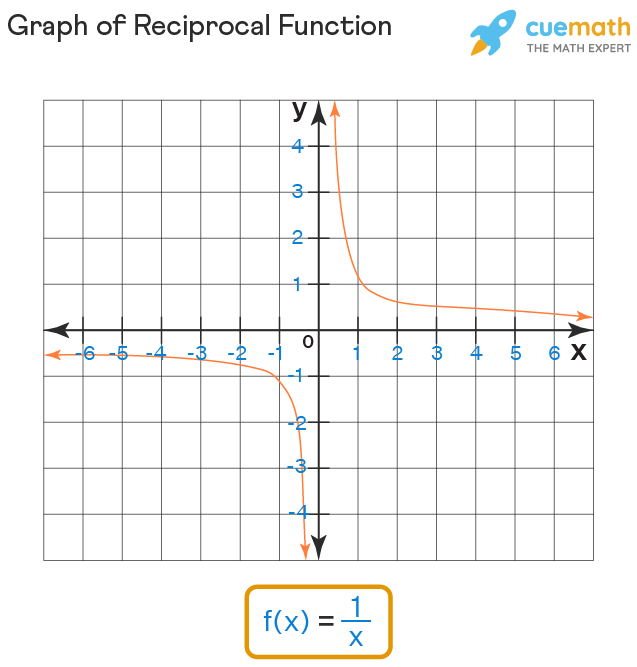
For a reciprocal function f(x) = 1/x, 'x' can never be 0 and so 1/x can also not be equal to 0. From the graph, we observe that they never touch the x-axis and y-axis. The y-axis is said to be the vertical asymptote as the curve gets very closer but never touches it. Also, the x-axis is the horizontal asymptote as the curve never touches the x-axis.
Domain and Range of Reciprocal Function
The reciprocal functions have a domain and range similar to that of the normal functions. The domain of the reciprocal function is all the real number values except values which gives the result as infinity. And the range is all the possible real number values of the function.
Domain is the set of all real numbers except 0, since 1/0 is undefined
{x ∈ R: | x ≠ 0 }
Range is also the set of all real numbers.
{x ∈ R: | x ≠ 0 }
How To Solve Reciprocal Functions?
The reciprocal functions of some of the numbers, variables, expressions, fractions can be obtained by simply reversing the numerator with the denominator. The method to solve some of the important reciprocal functions is as follows.
- Reciprocal of a Number: To find the reciprocal we divide the number, variable, or expression by 1. For example, reciprocal of 6 is 1/6
- Reciprocal of a Variable: The reciprocal of a variable 'y' can be found by dividing the variable by 1. For example, the Reciprocal of y is 1/y
- Reciprocal of an Expression: The reciprocal of an expression can be found by exchanging the positions of numerator and denominator. Examples are, Reciprocal of x/(x - 4) is (x - 4)/x.
- Reciprocal of a Fraction:: Reciprocal of a fraction can be obtained by flipping the places of numerator and denominator. For example, reciprocal of 5/8 is 8/5.
- Reciprocal of a Mixed Fraction: Reciprocal of a mixed fraction can be obtained by finding the improper fraction and then finding its reciprocal. For example,to find the reciprocal of \(\begin{align}3\dfrac{3}{4}\end{align}\), we find the improper fraction which is 15/4 and now find the reciprocal 4/15.
Important Notes
- For a function f(x), 1/f(x) is the reciprocal function.
- Reciprocal is also called the multiplicative inverse.
- The reciprocal function y = 1/x has the domain as the set of all real numbers except 0 and the range is also the set of all real numbers except 0.
- An asymptote is a line that approaches a curve but does not meet it. For the reciprocal function f(x) = 1/x, the horizontal asymptote is the x-axis and the vertical asymptote is the y-axis.
- The vertical asymptote is connected to the domain and the horizontal asymptote is connected to the range of the function.
☛ Related Topics
The following topics help in a better understanding of reciprocal functions.
- Types of Functions
- Inverse Function
- Domain and Range
- Graphing Functions
- Relation and Function
Examples on Reciprocal Function
go to slidego to slidego to slide

Have questions on basic mathematical concepts?
Become a problem-solving champ using logic, not rules. Learn the why behind math with our certified experts
Book a Free Trial Class
Practice Questions on Reciprocal Function
go to slidego to slidego to slide
FAQs on Reciprocal Function
What Is Reciprocal Function In Math?
A reciprocal function is a function that can be inverted. For the reciprocal of a function, we alter the numerator with the denominator of the function. The function of the form. The function of the form f(x) = k/x can be inverted to a reciprocal function f(x) = x/k.
What is the equation of reciprocal function?
f(x) = 1/x is the equation of reciprocal function. Here the domain can take all the values except the value of zero, since zero results in infinity.
What Is the Inverse of The Reciprocal Equation?
f-1(x) is the inverse of the reciprocal equation f(x). The domain and range of the given function become the range and domain of the reciprocal function.
Is a Reciprocal Function Continuous?
Yes, the reciprocal function is continuous at every point other than the point at x =0. The values satisfying the reciprocal function are R - {0}.
Is a Reciprocal Function Bounded?
Since the reciprocal function is uniformly continuous, it is bounded.
What Is the End Behavior of a Reciprocal Function?
The end behavior of a reciprocal function describes the value of 'x' in the graph approaching negative infinity on one side and positive infinity on the other side.
Is the Reciprocal Function a Polynomial?
Yes, a polynomial is self-reciprocal.
How Do you Find the Reciprocal of a Quadratic Function?
By factoring and finding the x-intercepts of a quadratic equation(It may be zero, one, or two) we can find the reciprocal of a quadratic equation.
How To Differentiate A Reciprocal Function?
The differentiation of a reciprocal function also gives a reciprocal function. The differentiation \(\dfrac{d}{dx}.{1}{f(x)} = \dfrac{-1}{x^2}\)
How To Integrate A Reciprocal Function?
The integration of a reciprocal function gives a logarithmic function. \(\int \dfrac{1}{x}\) gives log x + c.
What Is The Reciprocal Function of Trigonometric Ratios?
The reciprocal function of trigonometric ratios gives another trigonometric ratios. f(x) = 1/Sinx = Cosecx, f(x) = 1/Cosx = Secx, f(x) = 1/Tanx = Cotx.
Source: https://www.cuemath.com/calculus/reciprocal-function/
0 Response to "Prove Continuity of Reciprocal Function by Composites F1f2 F1f2"
Post a Comment